In today’s geometry lesson, you’re going to learn about the triangle similarity theorems, SSS (side-side-side) and SAS (side-angle-side).

Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher)
In total, there are 3 theorems for proving triangle similarity:
- AA Theorem
- SAS Theorem
- SSS Theorem
Let’s jump in!
How do we create proportionality statements for triangles? And how do we show two triangles are similar?
Being able to create a proportionality statement is our greatest goal when dealing with similar triangles. By definition, we know that if two triangles are similar than their corresponding angles are congruent and their corresponding sides are proportional.
AA Theorem
As we saw with the AA similarity postulate, it’s not necessary for us to check every single angle and side in order to tell if two triangles are similar. Thanks to the triangle sum theorem, all we have to show is that two angles of one triangle are congruent to two angles of another triangle to show similar triangles.
But the fun doesn’t stop here. There are two other ways we can prove two triangles are similar.
SAS Theorem
What happens if we only have side measurements, and the angle measures for each triangle are unknown? If we can show that all three sides of one triangle are proportional to the three sides of another triangle, then it follows logically that the angle measurements must also be the same.
In other words, we are going to use the SSS similarity postulate to prove triangles are similar.
SSS Theorem
Or what if we can demonstrate that two pairs of sides of one triangle are proportional to two pairs of sides of another triangle, and their included angles are congruent?
This too would be enough to conclude that the triangles are indeed similar. As ck-12 nicely states, using the SAS similarity postulate is enough to show that two triangles are similar.
But is there only one way to create a proportion for similar triangles? Or can more than one suitable proportion be found?
Triangle Similarity Theorems
Just as two different people can look at a painting and see or feel differently about the piece of art, there is always more than one way to create a proper proportion given similar triangles.
And to aid us on our quest of creating proportionality statements for similar triangles, let’s take a look at a few additional theorems regarding similarity and proportionality.
1. If a segment is parallel to one side of a triangle and intersects the other two sides, then the triangle formed is similar to the original and the segment that divides the two sides it intersects is proportional.
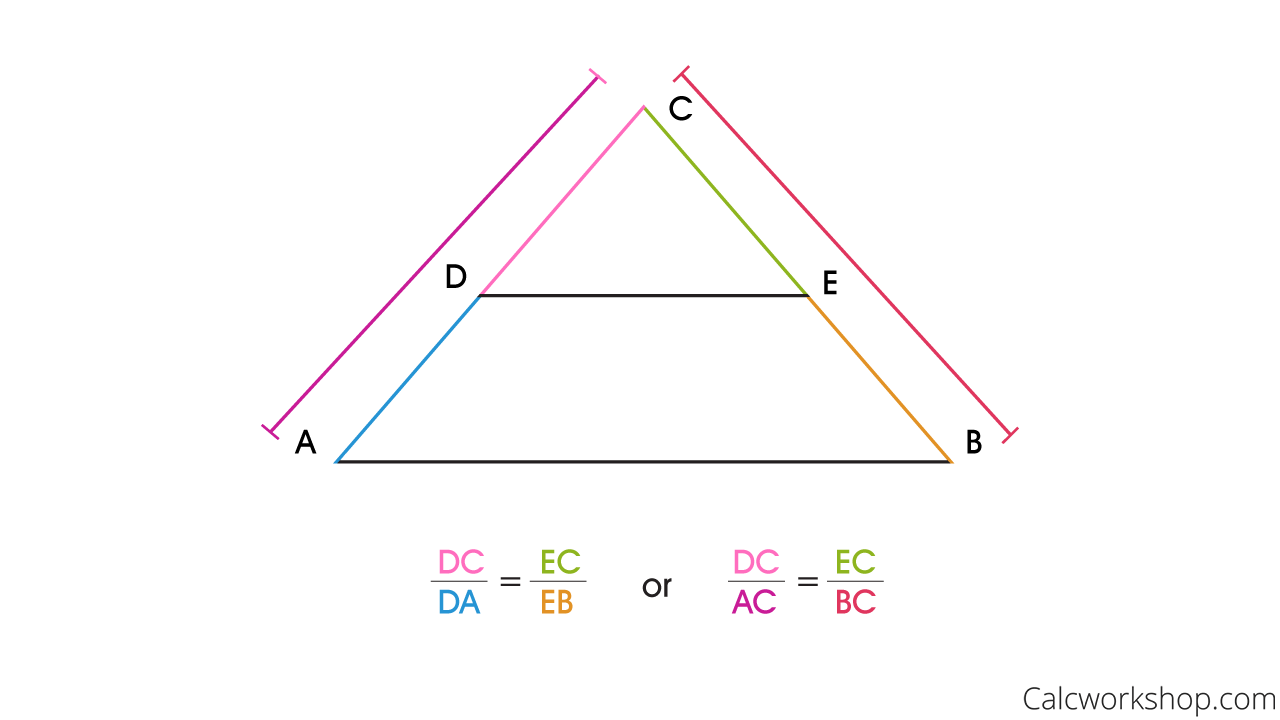
Proportional Segment Theorem
2. If three parallel lines intersect two transversals, then they divide the transversals proportionally.
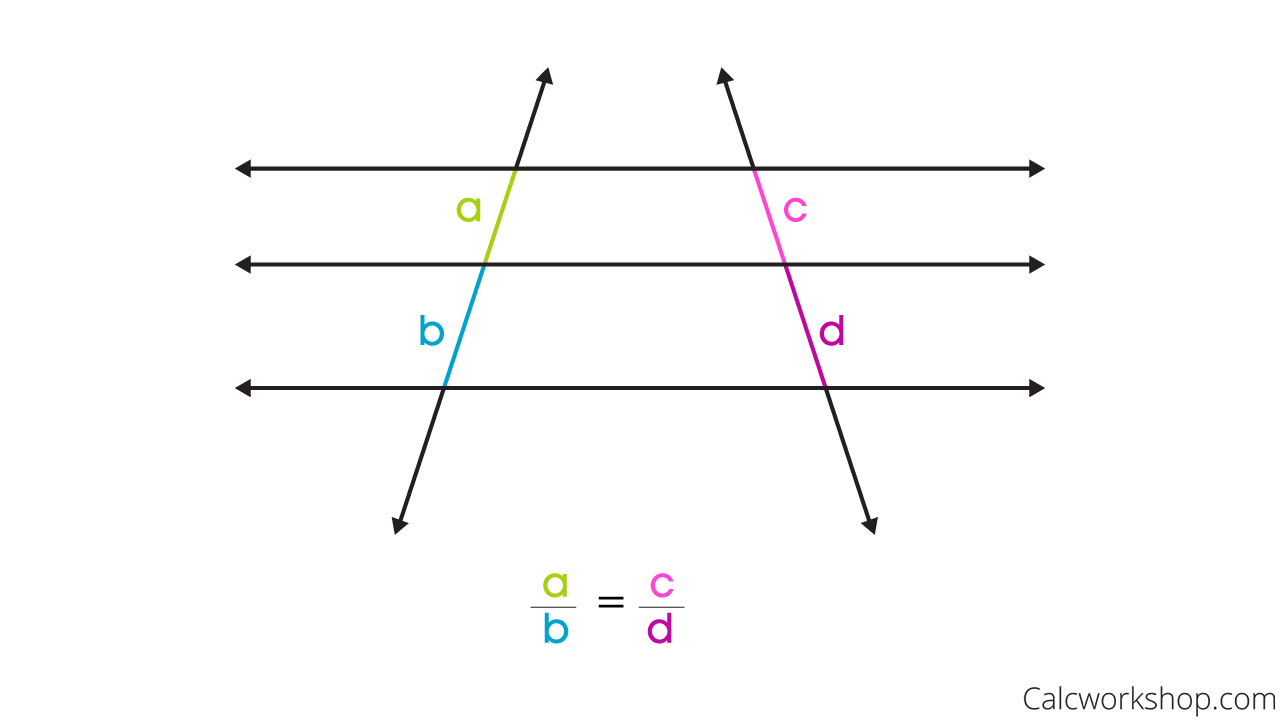
Proportional Transversal Theorem
3. The corresponding medians are proportional to their corresponding sides.
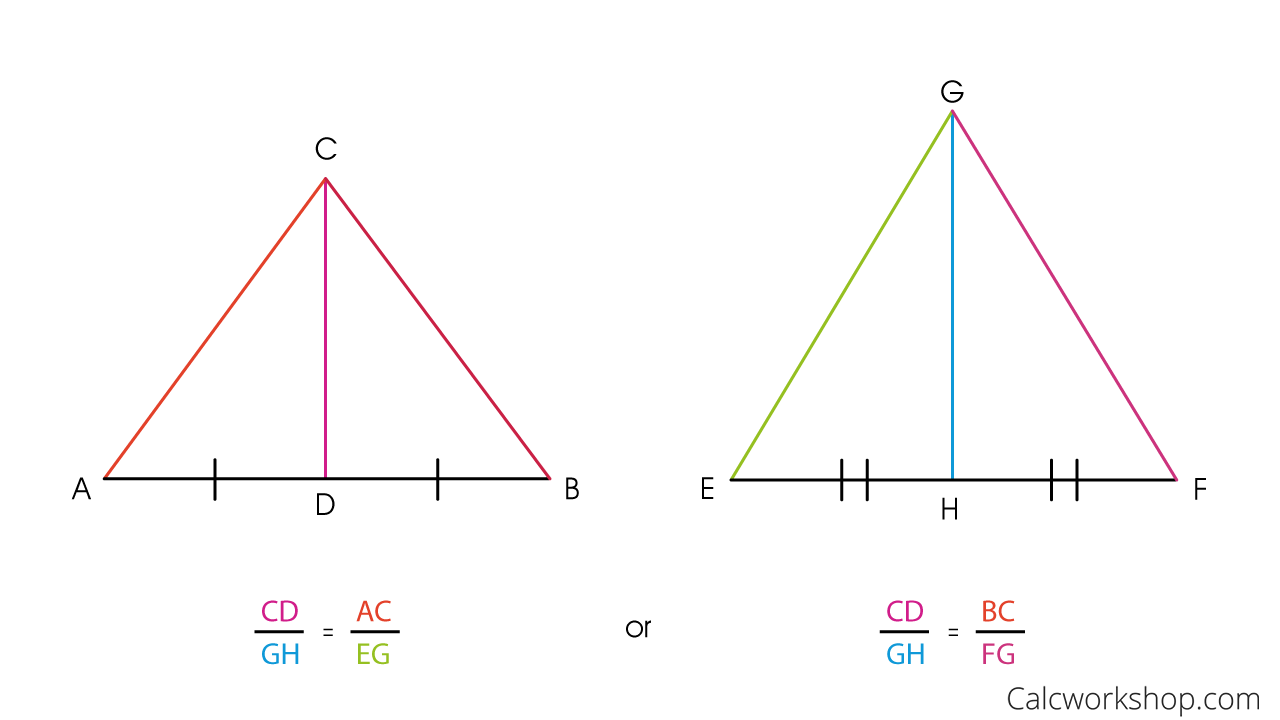
Corresponding Medians
4. If a ray bisects an angle or a triangle, then it divides the opposite side into segments whose lengths are proportional to the lengths of the other two sides.
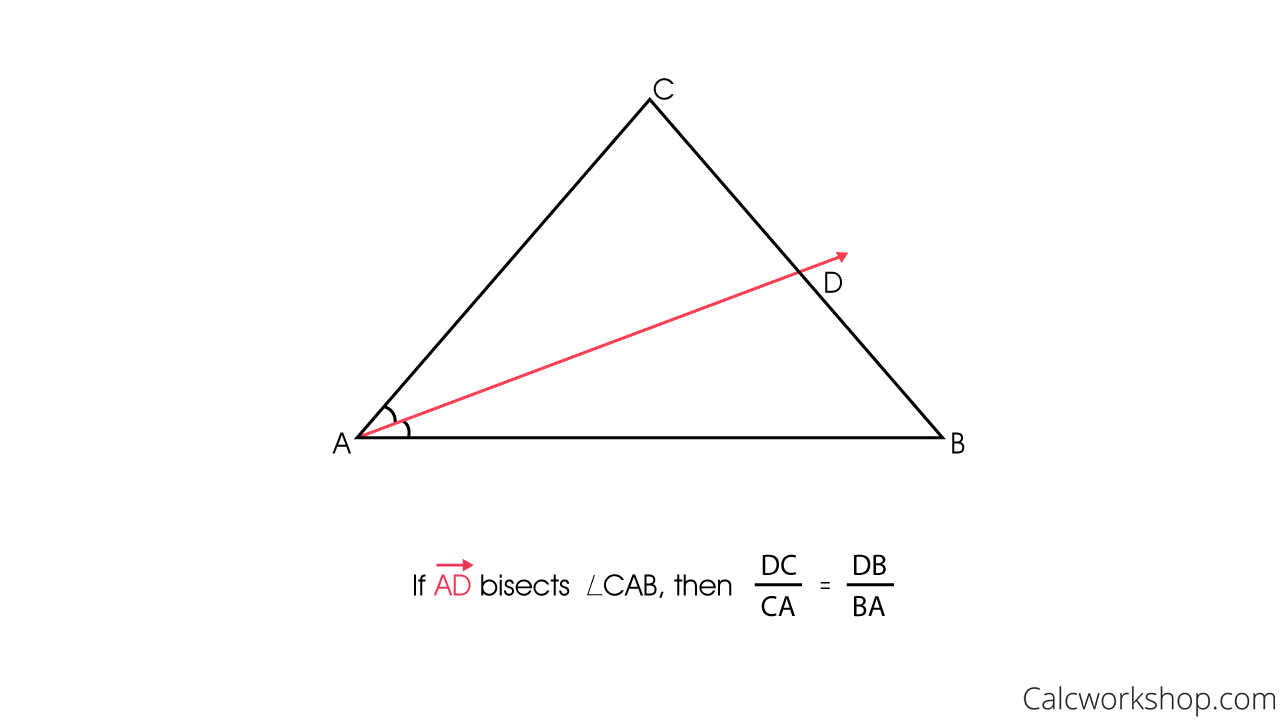
Ray Bisecting a Triangle Creating Proportional Sides
5. The perimeters of similar polygons are proportional to their corresponding sides.
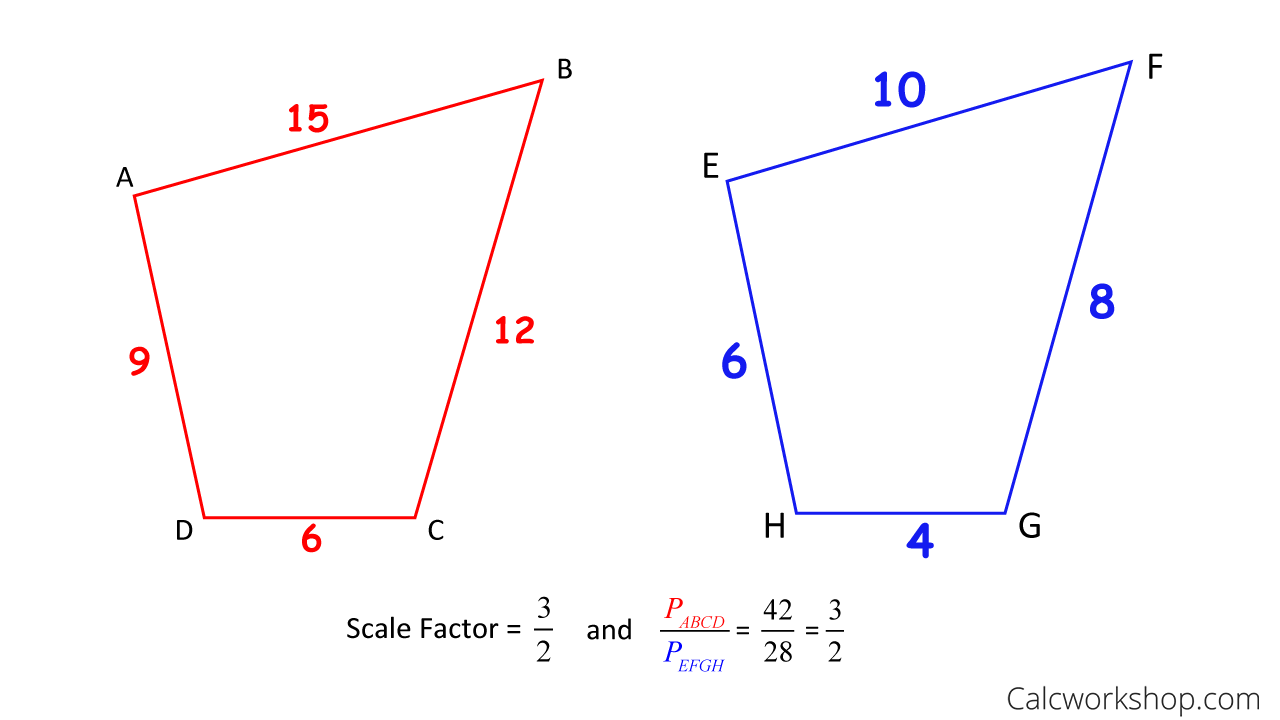
Perimeter of Similar Polygons
Together we are going to use these theorems and postulates to prove similar triangles and solve for unknown side lengths and perimeters of triangles.
Triangle Theorems – Lesson & Examples (Video)
1 hr 10 min
- Introduction SSS and SAS Similarity Postulates
- 00:00:19 – Overview of Proportionality Statements for Segments Parallel to a Side of a Triangle
- 00:15:24 – Find the value of x given similar triangles (Examples #1-6)
- 00:28:42 – Given three parallel lines cut by two transversals, find the value of x (Example #7)
- 00:31:36 – Overview of SSS and SAS Similarity Postulates and Similarity Theorems
- Exclusive Content for Member’s Only
- 00:35:37 – Determine whether the triangles are similar, and create a similarity statement (Examples #8-12)
- 00:51:37 – Find the unknown value given similar triangles (Examples #13-18)
- 01:02:36 – Find the unknown value or create the proportion for finding perimeter (Examples #19-21)
- 01:10:16 – Given similar triangles, find the perimeter (Examples #22-23)
- Practice Problems with Step-by-Step Solutions
- Chapter Tests with Video Solutions
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
Still wondering if CalcWorkshop is right for you?
Take a Tour and find out how a membership can take the struggle out of learning math.