Are you struggling with how to find interior angles of a polygon?

Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher)
We’ll you’re in the right place because that’s precisely what you’ll learn in today’s geometry lesson.
Let’s dive right in!
Interior Angles
Did you know that triangles play a critical role in finding the sum of the measures of the interior angles of any convex polygon?
Remember, a convex polygon has no angles that point inward, whereas a concave polygon makes something that looks like a cave where angles point toward the interior of the polygon.
And a regular polygon is one that is both equilateral (all sides are congruent) and equiangular (all angles are congruent).
Starting with any size polygon, let’s draw diagonals from one vertex. We will quickly notice that these diagonals help to divide the polygon into triangles. And the number of triangles we can create determines the sum of the interior angles.
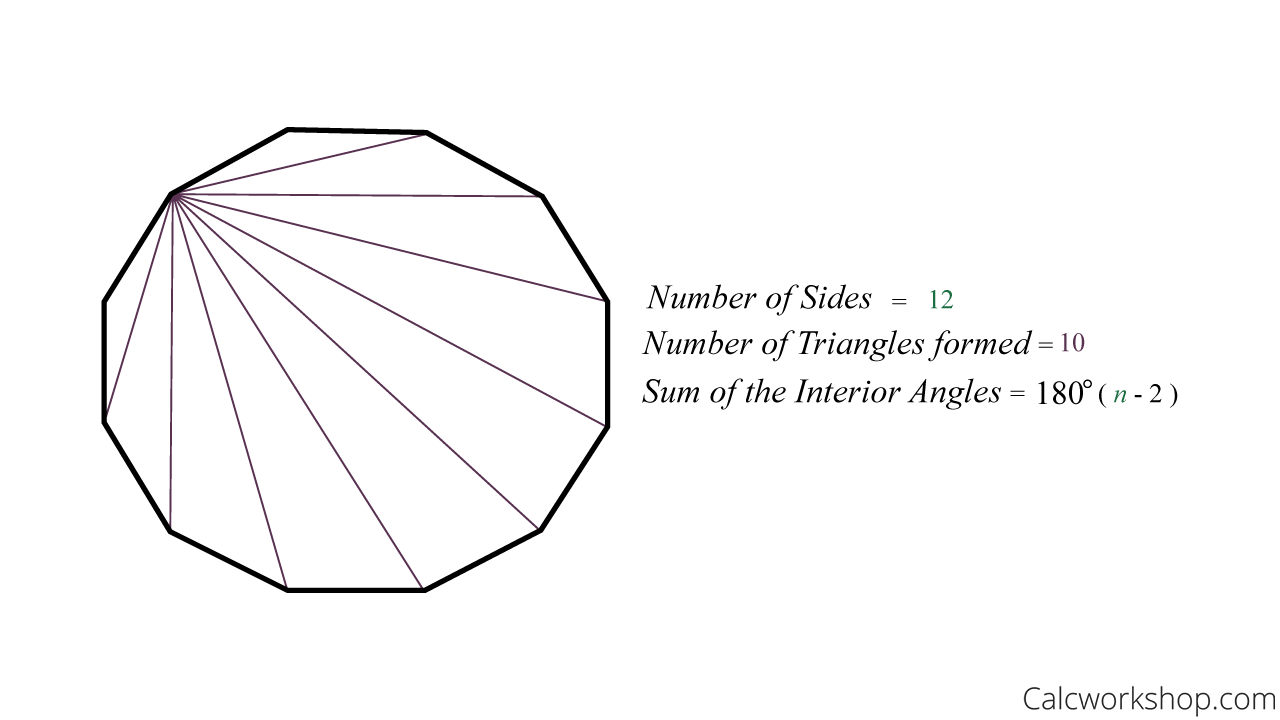
Sum of Interior Angles of a Polygon
How?
Since we know that the sum of interior angles in a triangle is 180°, and if we subdivide a polygon into triangles, then the sum of the interior angles in a polygon is the number of created triangles times 180°.
So, in general, this means that each time we add a side, we add another 180° to the total, as Math is Fun nicely states.
KEY FACT: To calculate the sums of the interior angles of any convex n-gon, use the general formula: 180Degrees*(n-2). (where n represents the number of sides of the polygon)
The chart below represents the formula for each of the most common polygons (triangle, quadrilateral, pentagon, hexagon, etc.).
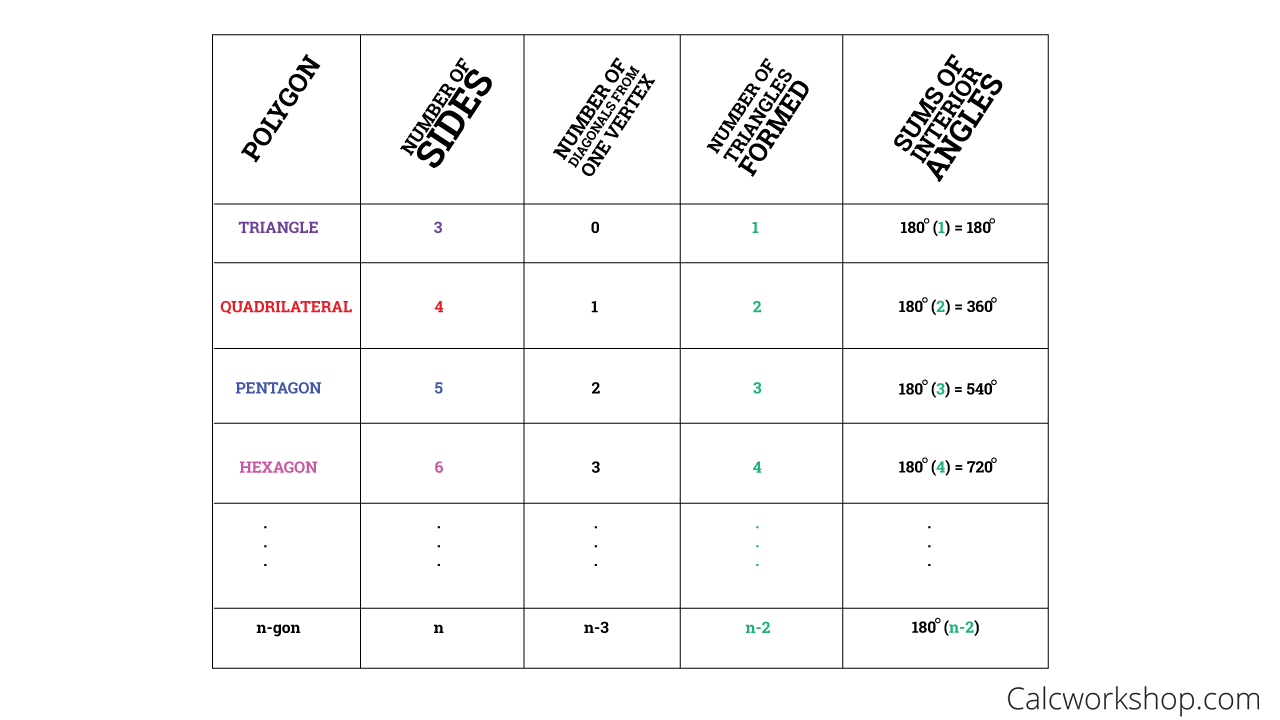
Polygon Chart
Additionally, if we have a regular polygon (i.e., all sides and angles are equal), then we can find the measure of each interior angle by dividing the sum of the interior angles by the number of sides. You’ll learn how to do this with the steps outlined in the video below.
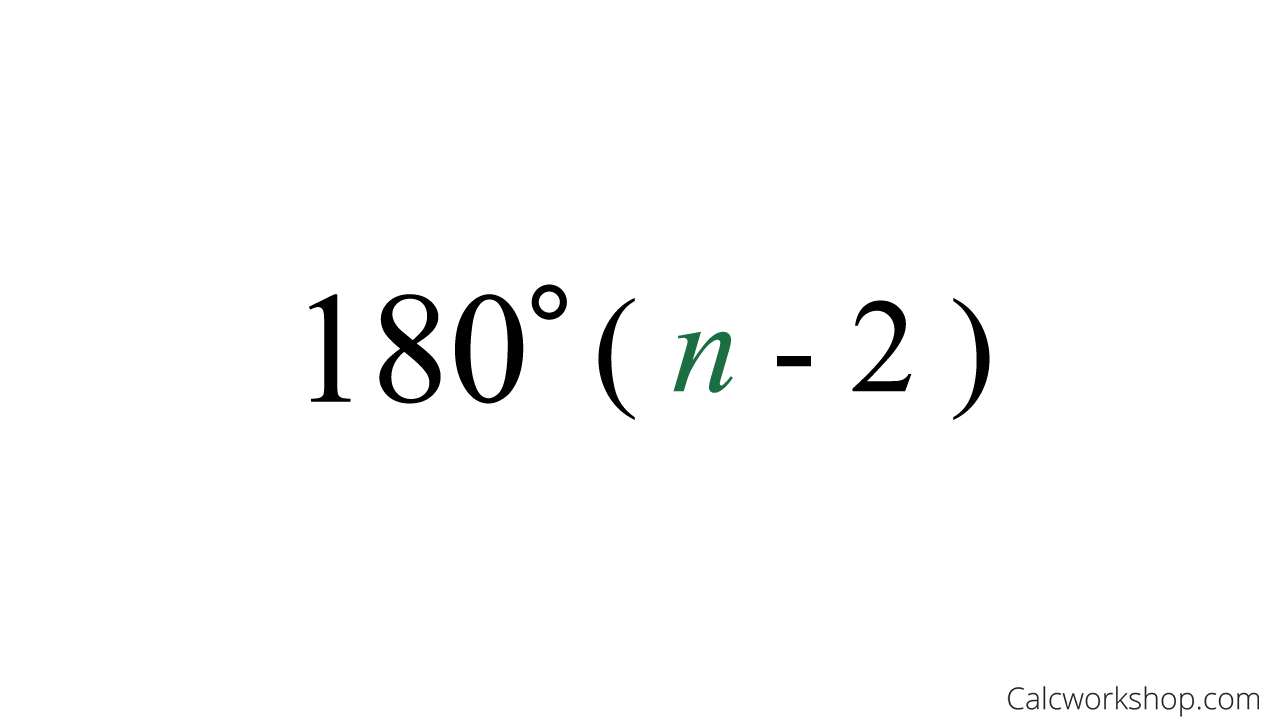
Interior Angle Formula
Exterior Angles
Moreover, did you know that the sum of the measures of the exterior angles, with one angle at each vertex, is 360°?
This means that if we have a regular polygon, then the measure of each exterior angle is 360°/n.
For example, if we have a regular pentagon (5 sided polygon with equal angles and equal sides), then each exterior angle is the quotient of 360 degrees and the number of sides as indicated below.
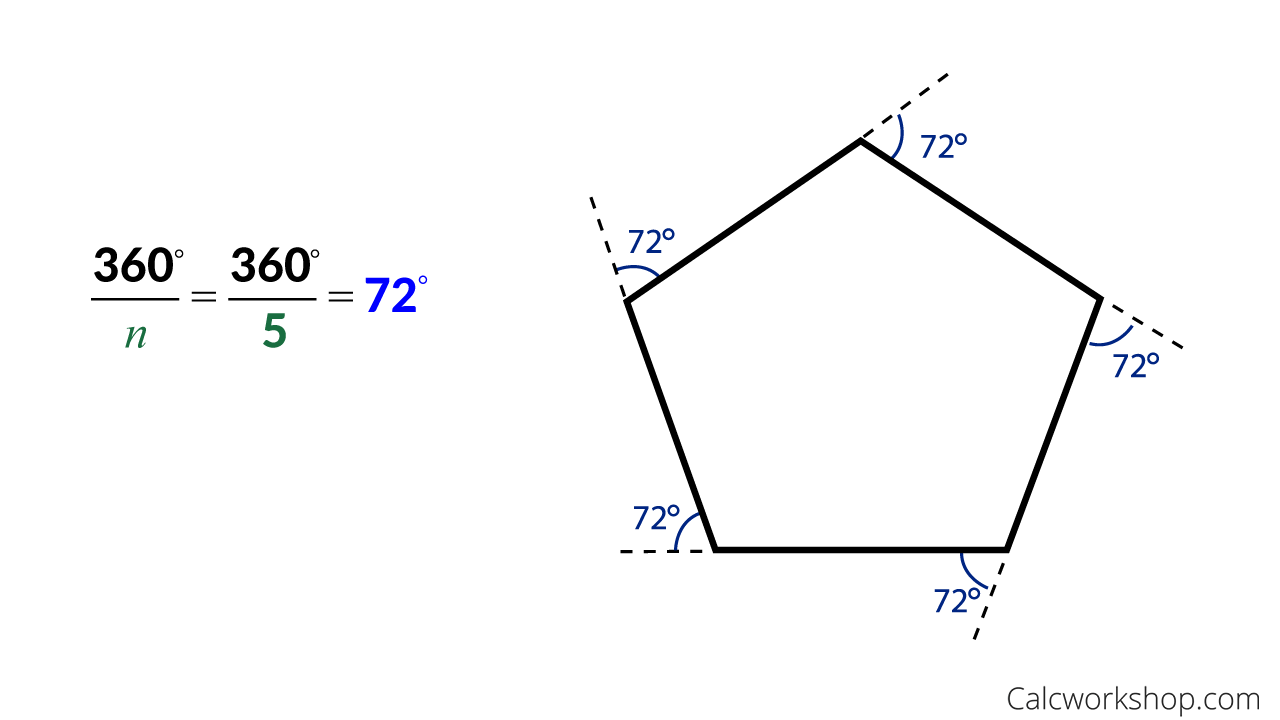
Sum of Exterior Angles
In the video below, you’ll learn how to:
- Find the sum of interior angles for various polygons.
- Find the measure of each interior and exterior angle for a regular polygon.
- Determine the number of sides a regular polygon has if you are given the measure of one exterior or interior angle.
- Find the measures of unknown angles for a polygon using our new formulas and properties.
Video – Lesson & Examples
46 min
- Introduction to Video: Angles of Polygons
- 00:00:35 – Formulas for finding the sum of the interior and exterior angles of a polygon
- Exclusive Content for Member’s Only
- 00:12:01 – Find the sum of the interior angles and the measure of each interior and exterior angle for a regular polygon (Examples #1-5)
- 00:23:37 – Find the number of sides of a regular polygon, given an exterior angle (Examples #6-8)
- 00:26:57 – Given an interior angle of a regular polygon find the number of sides (Examples #9-11)
- 00:33:54 – How to find interior angles of a irregular polygon (Example #12)
- 00:38:04 – Find all the unknown angles for the given polygon (Example #13)
- Practice Problems with Step-by-Step Solutions
- Chapter Tests with Video Solutions
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
Still wondering if CalcWorkshop is right for you?
Take a Tour and find out how a membership can take the struggle out of learning math.