What do you do with infinity?
Namely, what do you do when a definite integral has an interval that is infinite or where the function has infinite discontinuity?
Both of these scenarios are called Improper Integrals.
Now, an Improper Integral is a definite integral that cannot be computed using normal Riemann integral techniques, according to Wolfram Alpha. Therefore, we are going to have to develop ways of replacing infinite or undefined limits with finite values.
There are two types of Improper Integrals:
- Definition of an Improper Integral of Type 1 – when the limits of integration are infinite
- Definition of an Improper Integral of Type 2 – when the integrand becomes infinite within the interval of integration.
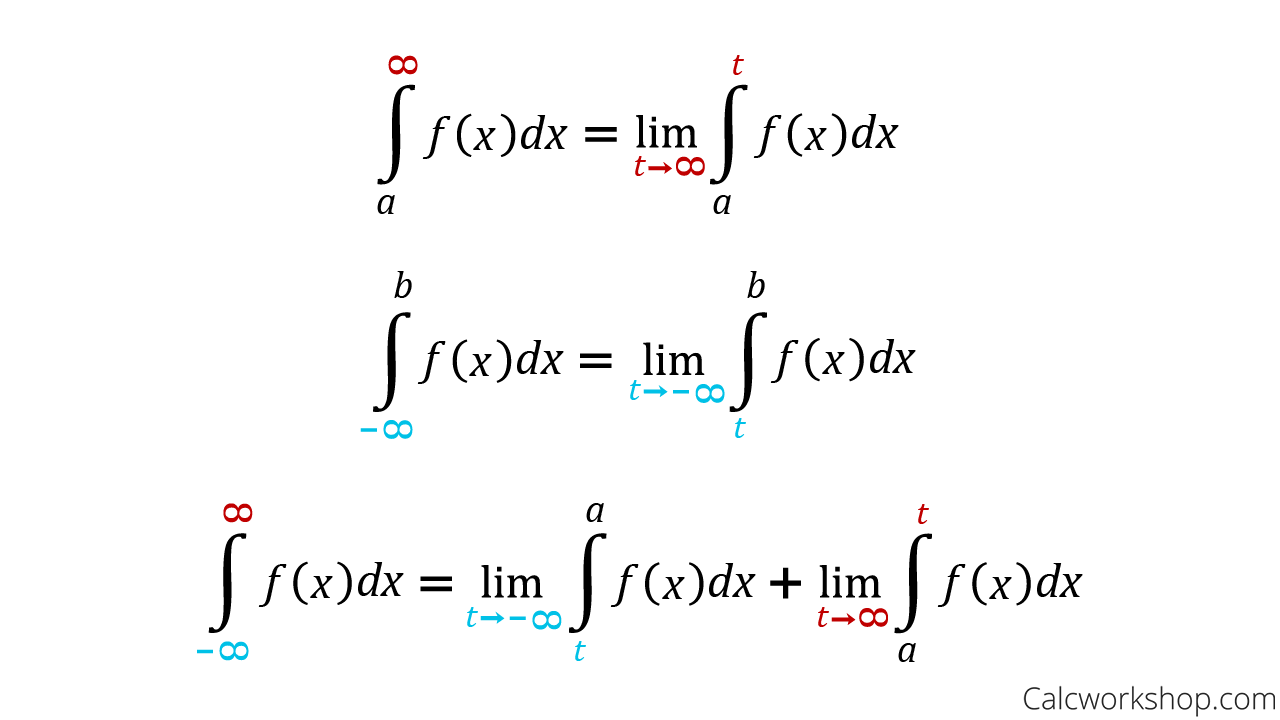
Changing Improper Integrals to Limits of Integrals
We will walk through five examples of Improper Integrals and see how we change our integral into a limit expression, which enables us to approach infinity and determine convergence and divergence.
Moreover, it is important to note that sometimes it is also beneficial to use the direct comparison theorem when dealing with improper integrals, as there are instances when the exact value of the improper integral cannot be found.
The idea behind the comparison test for improper integrals is to graph our integral function and then compare it with a graph of a known expression that is similar to the one we are asked to integrate.
Then graph our two functions, ensuring that our chosen comparison function is above, or on top, whereas our given function is underneath, or on the bottom.
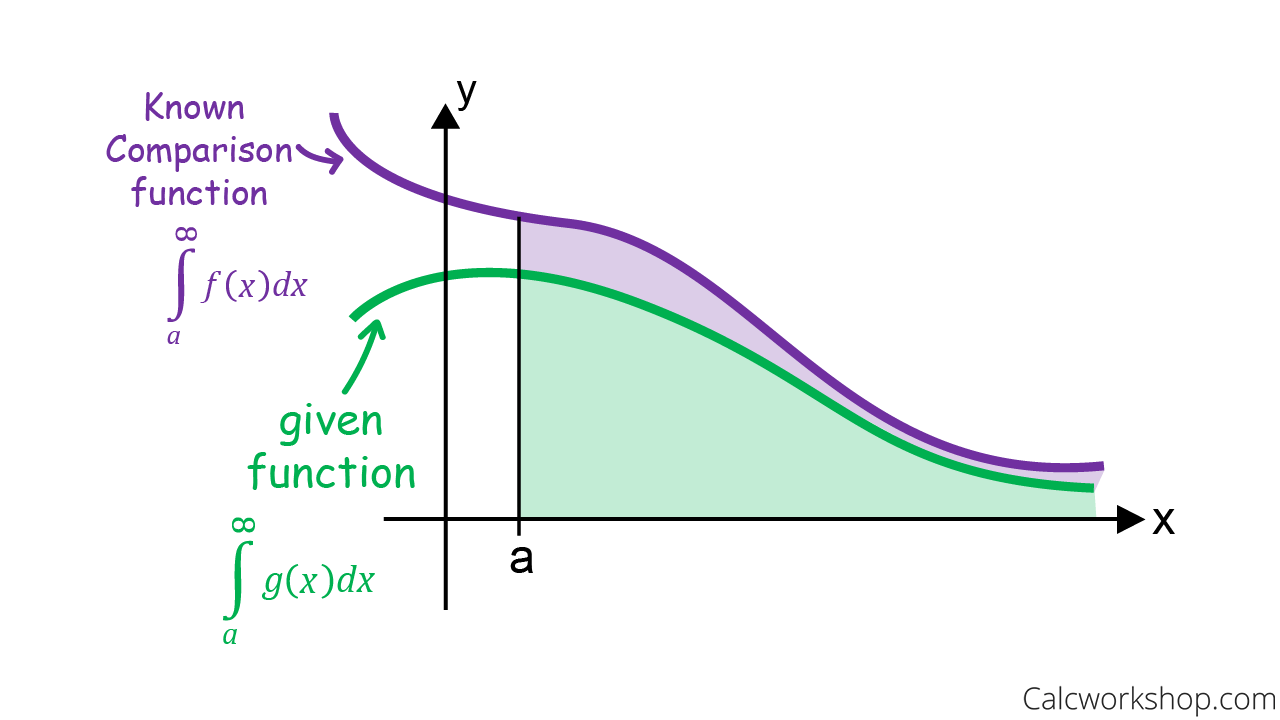
Comparison Theorem for Improper Integrals
Now we use the comparison theorem to determine convergence or divergence.
- If the area under the graph of our given function (the one on the bottom) is infinite than the area under the comparison function (the one on top) will also yield an infinite area. Therefore, both functions diverge.
- If we can show that the area under the comparison function (curve on top) is finite, then the area under our given function (curve on the bottom) is too; thus convergent.
We will go into much greater depth into the concept of comparison, and the comparison test, as it pertains to convergence in our sequences and series unit.
Improper Integrals Video
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
Still wondering if CalcWorkshop is right for you?
Take a Tour and find out how a membership can take the struggle out of learning math.