Have you ever wondered what the difference is between normal distributions and standard normal distributions?

Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher)
Or what the difference is between a z score and the standard deviation?
Well, if data tends to be around one central value then we say it is normally distributed. The spread, or distribution, has symmetry around the center or mean, and 50% of the data falls to the left of the center and 50% of the data falls to the right. And the standard deviation helps us to measure the spread or distance the data is from the mean.
We calculate the probability or the likelihood an outcome will occur, by determining the area under the normal distribution curve, or bell curve. The easiest way to find these values is by using the Empirical Rule, as we saw in our previous lesson. However, sometimes the data doesn’t cooperate, and we need to find the probability of an outcome that does not fall directly within 1, 2, or even 3 standard deviations from the mean.
So what do we do?
Z Scores!
The z score is used when we standardize a bell curve. First, we let the mean become zero (centered around the y-axis) and the z scores represent the standard score, similar to the standard deviations to the left and right of the mean. So a Z-score of 1 is 1 standard deviation above the mean and a Z score of -1 is 1 standard deviation below the mean.
In other words, a Z score is the number of standard deviations from the mean value and tells you where the scores lie on a normal distribution curve, as Statistics How To nicely states.
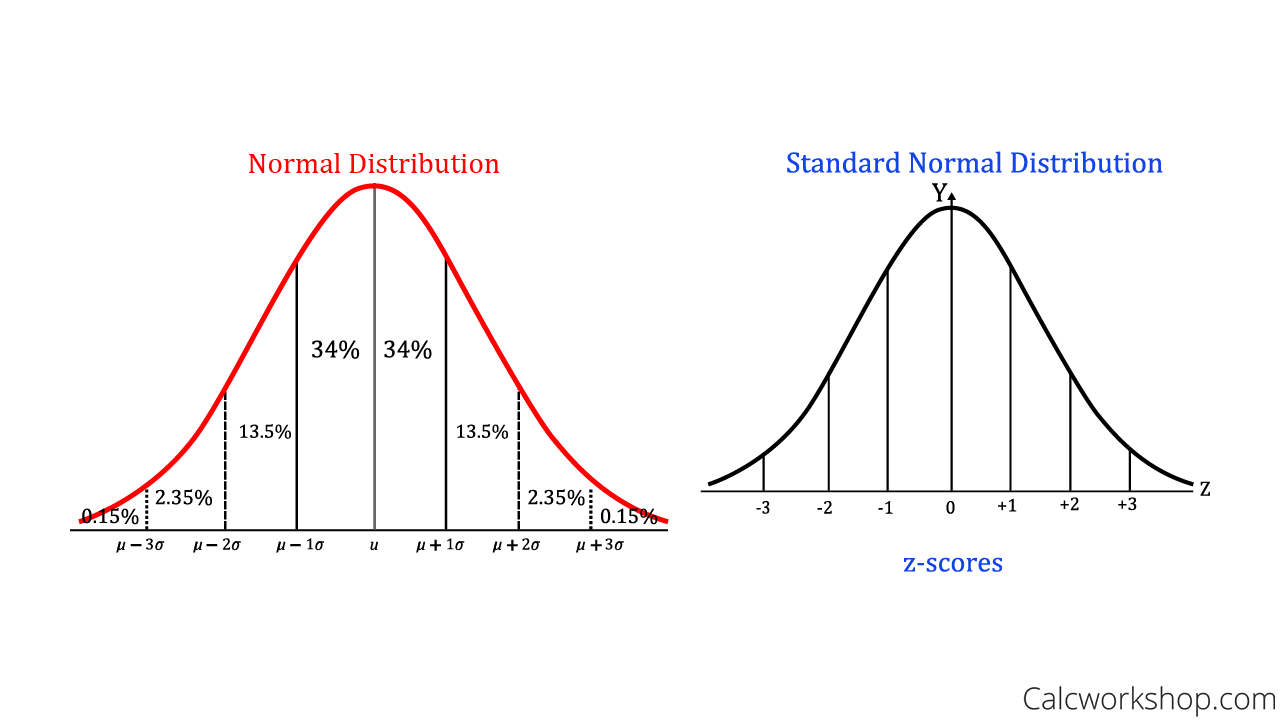
Standard Normal Distribution and Z Scores
Why do we need to standardize?
Rather than having to create a normal distribution curve for every possible dataset, we can just use one standard normal distribution curve and use set formulas to find probabilities.
But the real beauty behind z scores is found in how we calculate probability. As I already indicated, sometimes our data values don’t land perfectly at 1, 2, or 3 standard deviations from the mean, and we need to calculate precise proportions.
But the z score table provides us with accurate proportion values for all z score within -3.9 and +3.9 standard deviations from the mean. Therefore, without having to estimate or rely solely on the Empirical Rule, we can find the probability for any data set that is normally distributed.
But when using a z table, you need to keep in mind that the values provided in the table represent the probability at or below the indicated z score. Therefore, if you wish to find the likelihood of an outcome occurring after, or to the right, of the z-score you will need to use the fact that the entire area under the normal curve is 1 and subtract to find the desired probability or area.
Don’t worry, z scores and z tables are super easy to calculate and use!
Together we will learn how to find the z score for such scenarios as IQ score, the speed of a car, minimum ACT or SAT scores and cholesterol levels needed to be included in a study. We will also learn how to calculate the probability of students scoring within a range on a standardized test, the likelihood of finishing a race within a specified time, and the proportion of a population above or below a particular standard score.
Z Score (How-To) – Video
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
Still wondering if CalcWorkshop is right for you?
Take a Tour and find out how a membership can take the struggle out of learning math.