Do you ever get GCF and LCM confused?

Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher)
It happens, right?
Well, today, we’re going to learn the one method that gets you the answers to both very easily.
Let’s go!
But first let’s review the basic definitions of each.
What Is GCF And LCM
The Greatest Common Factor (also known as GCF) is the largest number that divides evenly into each number in a given set of numbers.
The Least Common Multiple (also known as LCM) is the smallest positive multiple that is common to two or more numbers.
Why Do You Need Both Methods
So will there ever be a time when we will need to use both the GCF, Greatest Common Factor and LCM, Least Common Multiple?
Yes, whenever we perform operations with fractions!
For instance, we may need to use the LCM to help us add two fractions, and also the GCF to simplify our result.
Consequently, you will need to know how to use both of these techniques at the same time.
How To Find GCF And LCM
How do you keep them straight and not mix them up?
Great question!
First, whenever you are asked to find both the greatest common factor and the least common multiple, always choose the prime factorization method, or the listing of prime factors, as it will save you time and is the only method that will work consistently.
And secondly, use the last letters of GCF and LCM to find what you need!
Here’s a trick: GCF = Fewer and LCM = More
Remember, when using our prime factorization technique, we choose the fewest common factors for the GCF, and for the LCM, we choose the most of each factor as discussed at Minnesota State University.
Example #1 — Two Numbers
Working a few problems will help to make sense of how this works.
For our first question, let’s find the GCF and find the LCM of two numbers: 12 and 18
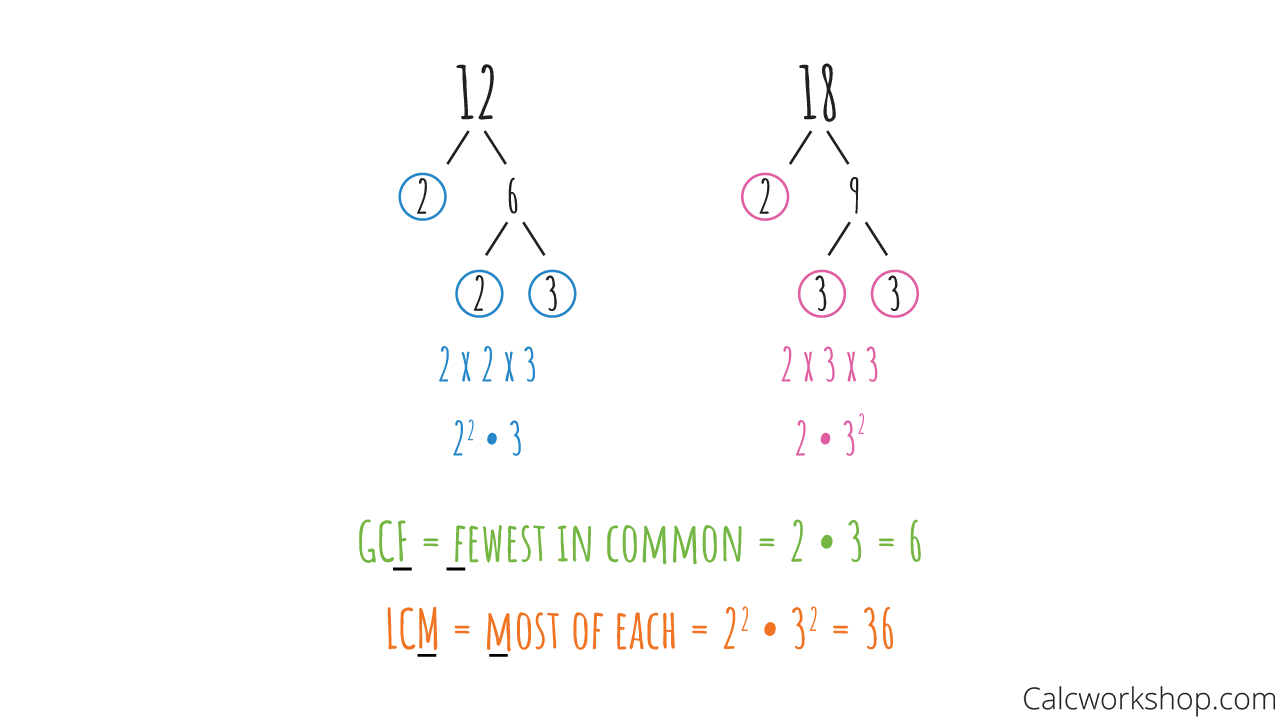
Find GCF and LCM of Two Numbers — Example
This means that the GCF of (12 and 18) is 6, and the LCM of (12 and 18) is 36.
Example #2 — Three Numbers
Now let’s work a problem involving three numbers.
Find the GCF and LCM of 15, 18, 24
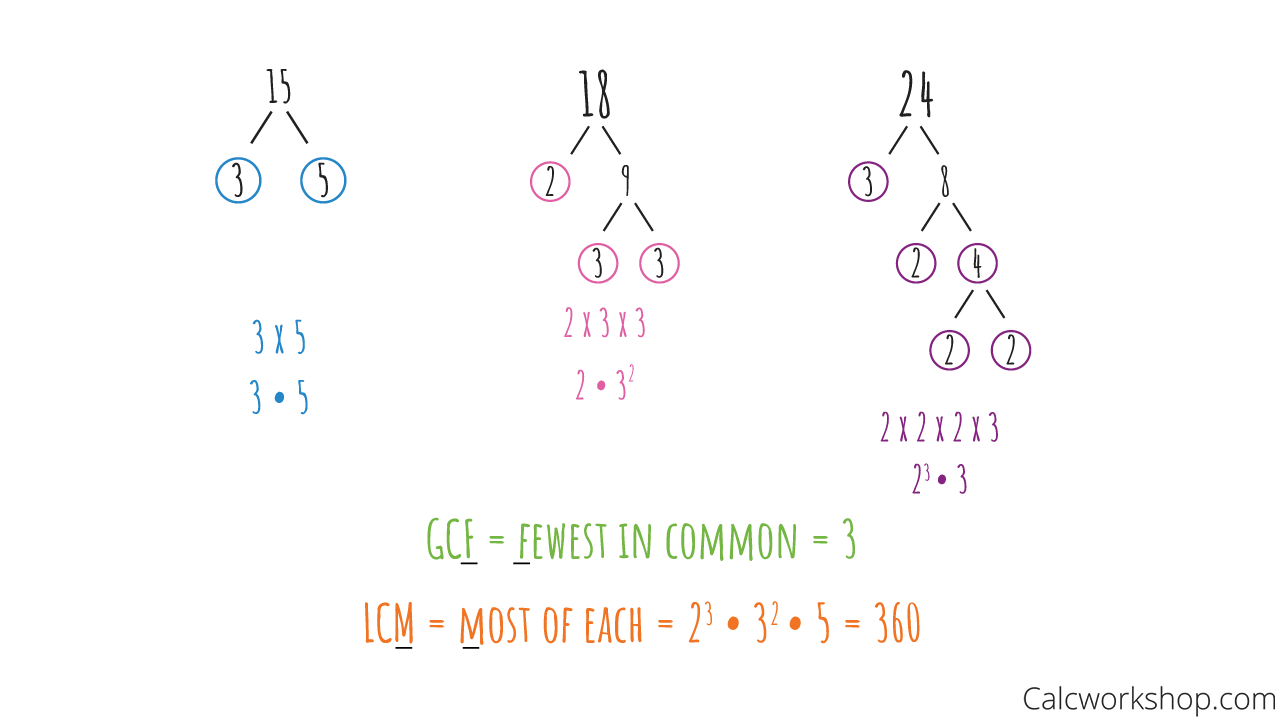
Find GCF and LCM of Three Numbers — Example
So…
- The GCF of (15, 18, and 24) is 3.
- And the LCM of (15, 18, and 24) is 360.
Using prime factorization and our trick for remembering what factors to choose is a snap!
Closing Thoughts
Now, I would like to point out that the phrase GCF has many synonyms. So, if you ever hear or see one of these alternate phrases, don’t be alarmed. Just know they all mean the same thing – find the greatest positive integer that divides evenly into two or more numbers.
The alternative terminologies for the Greatest Common Factor (GCF) are:
- Highest Common Factor (HCF)
- Greatest Common Divisor (GCD)
- Greatest Common Measure (GCM)
- Highest Common Divisor (HCD)
And while there are no alternate terminologies for Least Common Multiple, you will hear Least Common Multiple (LCM) and Least Common Divisor (LCD) used together quite often. Sometimes, they will be used interchangeably.
The LCM is how we find common multiples of two or more numbers, whereas the LCD is the least common multiple in a fraction’s denominator. So, the LCD is a subset or special case of the LCM. But in all honesty, they require the same math process, so many teachers and students use these two phrases as synonyms.
But, regardless of what the technique is called, the process for finding the greatest common factor and the least common multiple is very straightforward.
Worksheet (PDF) — Hands on Practice
Put that pencil to paper in these easy to follow worksheets — expand your knowledge!
GCF and LCM — Practice Problems
GCF and LCM — Step-by-Step Solutions
Video Tutorial — Full Lesson w/ Detailed Examples
Together we will work through various exercises involving two and three numbers to master the techniques of finding the GCF and LCM and never getting them mixed up.
30 min
- Introduction to Video: GCF and LCM
- 00:00:26 – How do you find the Greatest Common Factor and the Least Common Multiple?
- 00:01:45 – Find both the GCF and LCM (Examples #1-3)
- 00:14:17 – Determine the GCF and the LCM of three numbers (Examples #4-7)
- Practice Problems with Step-by-Step Solutions
- Chapter Tests with Video Solutions
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
Still wondering if CalcWorkshop is right for you?
Take a Tour and find out how a membership can take the struggle out of learning math.