Did you know that snap, crackle, and pop aren’t just the mascots of Kellogg’s Rice Krispies cereal but are the names of some higher order derivatives?

Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher)
It’s true!
Real Life Examples
A higher-order derivative means the derivatives other than the first derivative and are used to model real-life phenomena like most transportation devices such as:
- Cars
- Planes
- Rollercoasters
- Trampolines
In fact, if you’ve ever ridden around in a car, or better yet, experiencing the thrill of a rollercoaster, then you’ve physically experienced most of these types of derivatives.
Terminology
A function is called position or displacement, and the first derivative tells us how fast a function is changing over time. This means that the first derivative, or slope of a function, directly relates to a function’s velocity.
Similarly, the second derivative tells us how fast the first derivative is changing. That means we can look at how fast the slope is changing. This is called acceleration.
Likewise, the third derivative, sometimes called the jerk, is the change in acceleration. If you picture yourself riding in a car and the driver suddenly accelerates or quickly slams on the breaks, your body will feel a “jerk” in that direction, hence, its name.
Equally, with the change in acceleration, you will also lead to a snap, crackle, or pop as they are the rate of change with respect to time of the jerk, snap, and crackle, respectively, as the European Journal of Physics accurately states.
Thankfully we don’t feel these snaps, crackles, or pops while riding in a car or on a rollercoaster because cars and rollercoasters, while designed to give us a sensory feeling, are designed to reduce harm and discomfort.
But, if you were unfortunate enough to find yourself in a car or rollercoaster where the safety regulations have been removed, then your body and bones may snap, crackle, and pop as the speed and force of vibrations increase.

Higher Order Derivative Names
Now while the names probably elicit a laugh or at least a smile, I should point out that only the first three names for higher order derivatives (velocity, acceleration, and jerk) are used with any consistency.
How To Find Higher Order Derivatives
The key to finding derivatives beyond the first order is to just keep taking the derivative!
Example – Fourth Degree Polynomial
For practice, let’s find the 5th derivative for the following function.

Degree 4 – Polynomial Equation
First, we will find the velocity or the first derivative by using our differentiation rules to obtain

First Derivative – Velocity
Now we will differentiate this new equation to find the second derivative or the acceleration.

Second Derivative – Acceleration
Next, we will differentiate this new equation again and obtain the third derivative, called the jerk.

Third Derivative – Jerk
We can differentiate again to obtain the fourth derivative to be

Fourth Derivative – Snap Jounce
And continuing, we will differentiate again to find the fifth derivative.

Fifth Derivative – Crackle Flounce
And that’s all there is to it. We just keep taking derivatives.
But can we keep taking derivatives forever?
Sure, but if you are given a polynomial of degree n, then the derivative will eventually become zero after the n+1 derivatives, as Paul’s Online Notes so nicely explains.
You just witnessed this yourself in the example above. We started with a fourth-degree polynomial, and at the fifth derivative, it went to zero.
Infinitely Differentiable
However, some functions are infinitely differentiable! That means you can keep taking the derivative of the function forever and ever.
I like to think of this process as descending (going down) a ladder or staircase. Each derivative we take is a step further down the ladder.
Example – Trig Equation
For this question, let’s find 4th derivatives for y = cos2x.
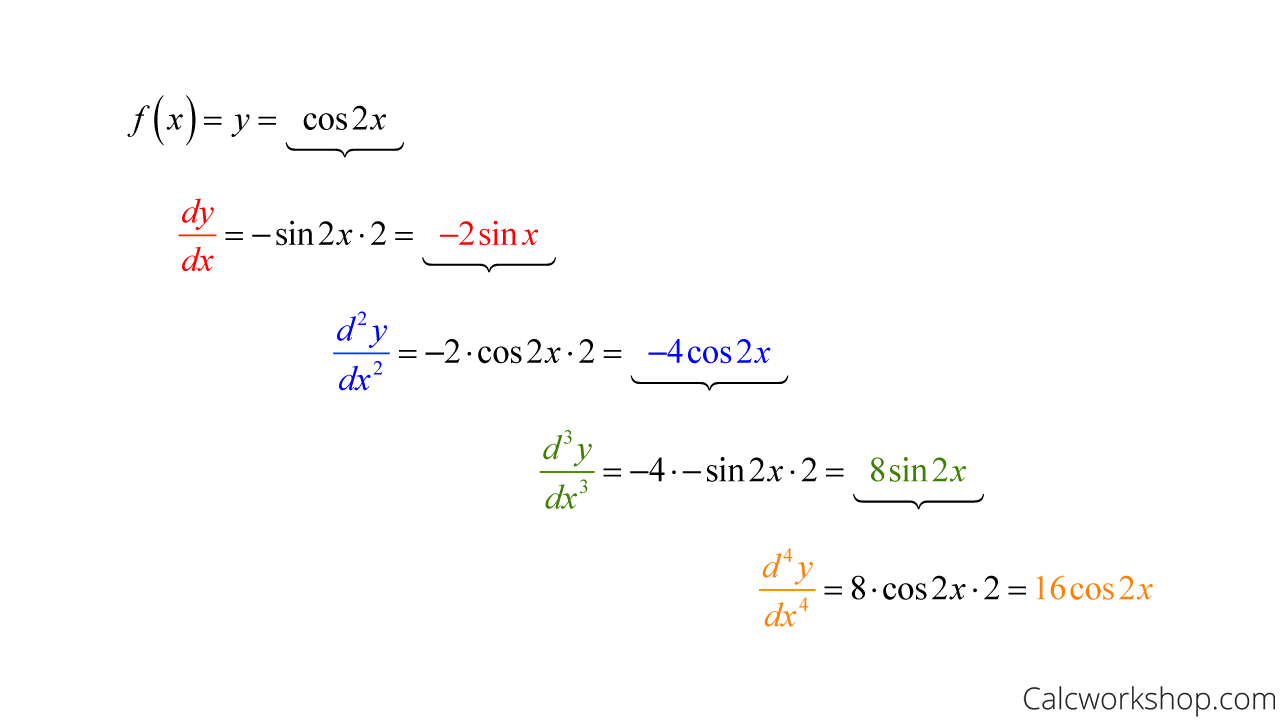
Find The Higher Order Derivative Of A Trig Function
While each derivative is continually different, we can see a cyclical pattern.
Wild!
So, together we will walk through 12 step-by-step examples, all while utilizing our previously learned differentiation techniques. And see how higher-order derivatives empower us to make real-life connections to engineering, physics, and planetary motion.
Video Tutorial w/ Full Lesson & Detailed Examples (Video)
Boost Your Calculus Scores with Step-by-Step Instruction
Jenn’s Calculus Program is your pathway to confidence. Each lesson tackles problems step-by-step, ensuring you understand every concept.
No more knowledge gaps – Jenn’s instruction bridges the missing pieces, so you’re always in stride with your class.
Calculus won’t block your academic or professional goals. Lay a solid foundation, one lesson at a time.
Your path to calculus success is just one click away.
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
Still wondering if CalcWorkshop is right for you?
Take a Tour and find out how a membership can take the struggle out of learning math.