We know that a system is an interdependent group of items that are working together, but what does it mean to solve a system?

Jenn, Founder Calcworkshop®, 15+ Years Experience (Licensed & Certified Teacher)
Well, solving a system of linear equations is about finding what all of the equations have in common.
In other words, we are trying to find the point of intersection!
Now there are several ways for us to solve a system of equations to find the intersection point, and this lesson is our first method – Solving Systems of Equations by Graphing.
To solve a system of linear equations by graphing we simply graph both equations in the same coordinate plane, as Math Planet accurately states, and we identify the point where the two lines intersect.
In fact, the whole graphic method process can be boiled down to three simple steps:
- Transform both equations into Slope-Intercept Form.
- Sketch the graph of each linear equation in the same coordinate plane.
- Determine the solution of the system.
If the lines intersect, the coordinates of the point of intersection give the solution to the system.
If the lines are parallel, there is no solution.
If the lines coincide, meaning they are the same line, there are an infinite number of solutions.
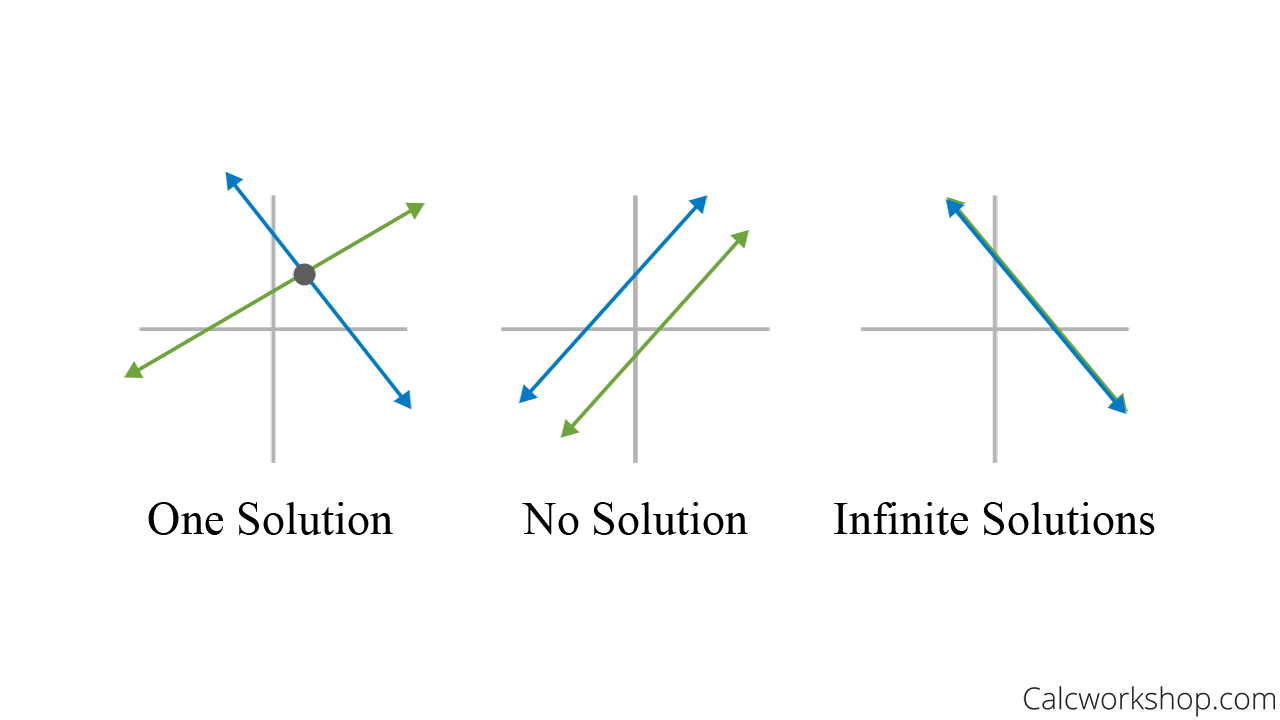
Solutions for a System of Equations
If a system has one or an infinite number of solutions, then it is considered a consistent system. If there are no solutions, then it is deemed inconsistent.
Remember that in a system of two equations each equation contains two variables, x and y. This means that a system of two equations imposes two conditions on the variables at the same time, meaning we are looking for when both equations have the same x value and the same y value at the same moment. This is why systems of equations are also called simultaneous equations.
While the steps for solving systems graphically are easy to follow, the process does have some pitfalls.
Graphing by hand isn’t very precise, and it can be tedious. Therefore, we tend only to use the method of solving by graphing when we can employ a graphing calculator, as the other methods such as substitution, elimination, and row reduction are infinitely more accurate and efficient.
But as it’s important to visually understand what is happening when we solve a system (i.e., a picture is worth a thousand words) beginning our unit on solving systems by graphing is the logical first step.
Graphing Method (How-To) – Video
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
Still wondering if CalcWorkshop is right for you?
Take a Tour and find out how a membership can take the struggle out of learning math.